How To Measure a Bond’s Interest Rate Risk? Enter Duration, DV01 & Convexity

As interest rates rise, bond prices typically fall, and as rates fall, bond prices rise. Duration helps investors quantify this relationship. Bond duration is a measure of the sensitivity of a bond's price to changes in interest rates. It provides an estimate of the potential impact of interest rate fluctuations on the value of a fixed-income investment.

From Duration, we can derive a very practical and useful measure called DV01: this represents the change in the price of a bond for a 1 basis point (0.01%) change in yield. It is a useful metric for assessing interest rate risk in fixed-income securities, similar to bond duration. For example, for an investor who has a long bond position, with a DV01 of $10,000, if the interest rate moves from 5.00% to 4.99%, his P&L (Profit & Loss) has increased by $10,000.

The problem with Duration and DV01 is that they are a helpful predictor of P&L for normal, standard, moves in interest rates; however, when markets become volatile and the moves are large, we also need to take into account a bond’s convexity to determine our P&L.  Convexity measures the curvature of the bond price-yield curve. It represents the rate at which the bond's duration changes as interest rates change. While duration gives an estimate of the linear price-yield relationship, convexity helps account for the non-linearities. This leads to the following P&L approximation:

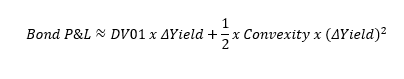
Understanding convexity is important for investors and portfolio managers seeking a more comprehensive understanding of interest rate risk in fixed-income securities. While duration provides a linear approximation, convexity adds a non-linear dimension, particularly relevant for managing the risk associated with interest rate changes.